" On the left is the nanocrystalline sample generated by a new algorithm developed in the computational materials science group. The "digital" sample has 200 grains with average grain size of 6 nanometers. This algorithm captures the features of poly-crystalline materials with correct grain boundary structure, size, and other topological characteristics. We are currently carrying out large scale molecular dynamics simulation and finite element modeling to investigate thermodynamic, transport, magnetic and mechanical properties of nanocrystalline materials."


Multiscale Simulation of Deformation and Fracture in Amorphous Materials

" On the left is the fractography of an amorphous metal undergoing deformation and fracture. The radiating lines from the semi-circular notch are shear bands. We are currently carrying out multiscale simulations, atomistic, mesoscopic and continuum, to try to understand how and why the deformation occurs in amorphous solids, which do not contain any extended defects such as dislocations as in crystalline materials. "


Mechanics on Mesoscales-Effects of Size and Interfaces

" On the left is the snapshot of a crack during its initial growth. The white areas are deformed regions. To capature the deformation process which is usually on the mesoscopic scales and strongly dependent of microstructures, we are developing a new approach using phase field model. Our goal is to incorporate various microstructures into the mechanics and capture the characteristics, such as branching, shear banding, dislocation and grain boundary interaction, and deformation in composites, during deformation and fracture. "



Phase Separation and Pattern Formation in Multicomponent Systems

" On the left is the snapshot of two phases, the black and the grey region, separated from a homogeneous phase of Ising spins using Monte Carlo method. The unique feature is the underlying structure, which is a Sierpinski carpet without translational symmetry. We are interested in how the topology of the underlying structure, and composition of multicomponent systems affect the kinetics and thermodynamics of phase separation."



Glass Transition and Non-Equilibrium Thermodynamics

" On the left is the snapshot of a liquid-like cluster in a glass-forming liquid which we found in the simulations using molecular dynamics method. This ramified structure is observed at temperatures close to the glass transition. The inhomogeneous and increasingly long-lived "patches" or "islands" in a supposedly homogeneous liquid is intriguing - is undercooled (metastable) liquid different from the liquid in equilibrium? We are currently investigating how the clusters, like the one shown on the left, contribute to the glass transition and how they affect dynamic and transport properties of glass-forming liquids."



Electromigration and Damages in Polycrystalline Cu and Al Metals

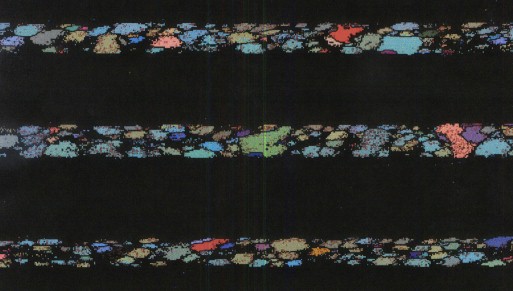" On the left is the orientation map of polycrystalline Cu wires used as interconnecting lines in most microelectronic devices (courtesy of K. Rodbell, IBM T. J. Watson Research Center). The large electrical current, ~ million Am per square centimeter, passing through these wires, causes metal atoms to migrate. As a result, voids and extrusions form in the thin wires and films, causing device failure. As device miniaturization requires smaller and smaller cross-sections, the electromigration induced failure becomes a serious issue for microelectronic industry. We are currently developing atomistic models and algorithms to model and simulate this phenomenon. In particular, we are interested in microstructure effect (grain size, orientation, grain boundary, solute, and second phase) on mass transportation caused by electromigration in polycrystalline metals. "


Melting and Crystal-to-Glass Transition

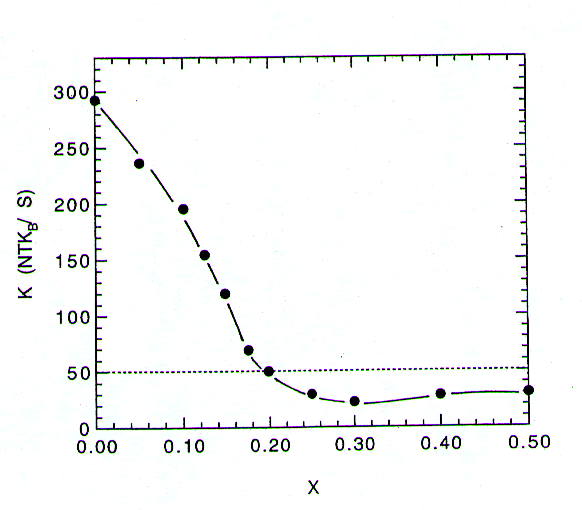" On the left is the dislocation coupling constant, or Kosterlitz-Thouless constant, versus disorder from our calculations. As predicted by theory, melting should occur when the coupling constant decreases to a critical value, 16pi, where two tightly bonded dislocations start separating. However, when temperature is high such as in thermal melting, dislocations do not have to unbind and become singlets; instead they can move together to form even more complicated defects such as grain boundaries. Our simulation shows that if the mobility of dislocations is reduced, the coupling constant indeed reaches the universal value; as a result, dislocation pairs separate into isolated singlets, resulting in a continuous melting transition with the formation of the so-called hexatic phase. We currently extend this work to three dimensions (3D) to search for mechanisms of melting and crystal-to-glass transition. An interesting and challenging task is to characterize dislocations in 3D using atomistic simulation when a large number of dislocations are present in the system. "


Algorithm and Methodology Development

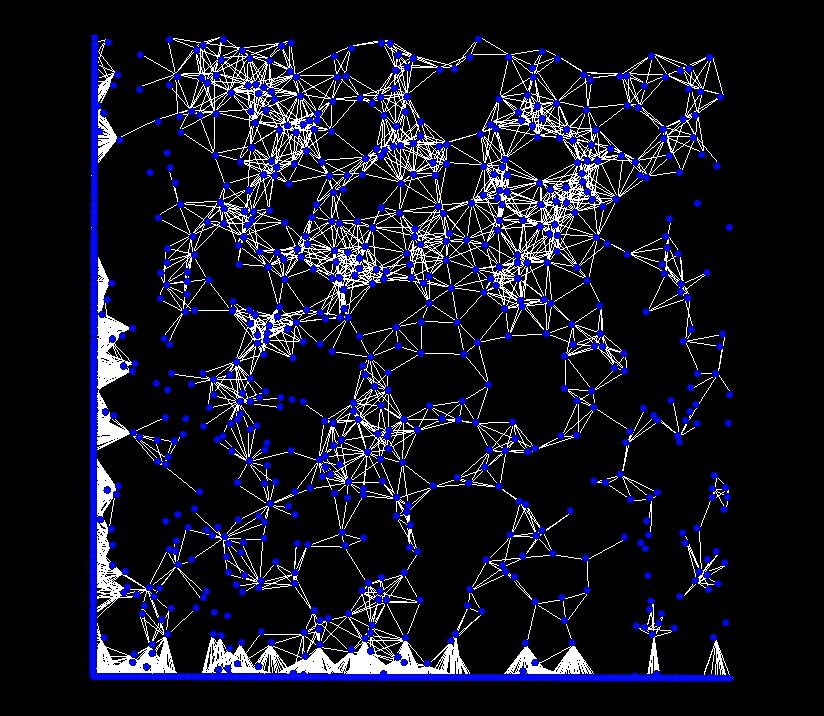" In order to simulate and model physical properties and processes in highly disordered systems and non-equilibrium processes, unavoidably we need to develop various new algorithms and simulation methods. (Most of the simulation algorithms available now are for equilibrium systems.) The following are some of the highlights in our algorithm development: (1) We developed a general algorithm that can reproduce subtle microstructural properties of nano- and poly-crystalline materials; (2) We found a new way to quench hard sphere liquids; (3) We developed an algorithm to calculate cluster lifetime for nucleation and glass transitions; (4) We developed a new method to compute free volumes in disordered systems. "